https://www.geogebra.org/m/nsnhvgcp
Based on https://www.geogebra.org/m/ttjsaabd
Κατηγορία: Γεωμετρία
Τετράπλευρο σε τετράπλευρο Διερευνητική εργασία
Τετράπλευρο σε τετράπλευρο Περιγραφή:
Δίνεται το τετράπλευρο ΑΒΓΔ, όπως στο σχήμα.
Αν Ε, Ζ, Η, Θ μέσα των αντιστοίχων πλευρών του, τότε:
α) Βρείτε όσες περισσότερες παρατηρήσεις μπορείτε
β) Αποδείξτε ή απορρίψτε όσες από τις παρατηρήσεις γράψατε στο προηγούμενο ερώτημα.
Πρoστατευμένο: Γεωμετρικοί Μετασχηματισμοί I.Yaglom
omilos1021b10122021 Δύναμη Σημείου ως προς κύκλο και άλλα προβλήματα
Τετράεδρο σε κύβο
https://www.geogebra.org/calculator/ttmkxkws
Πόσο χαμηλά πέφτει;
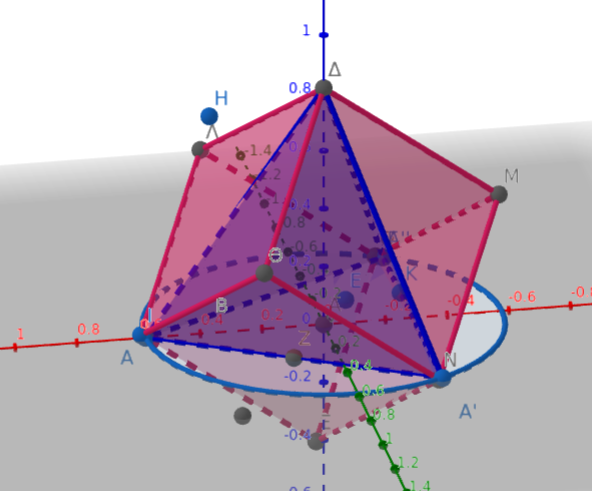
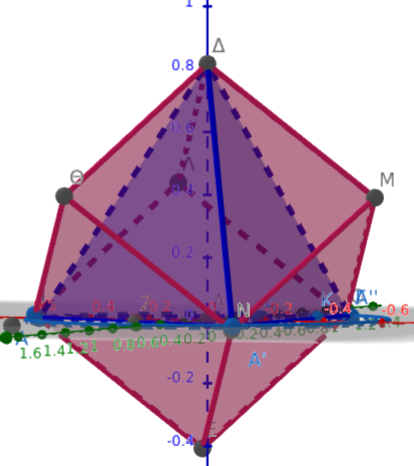
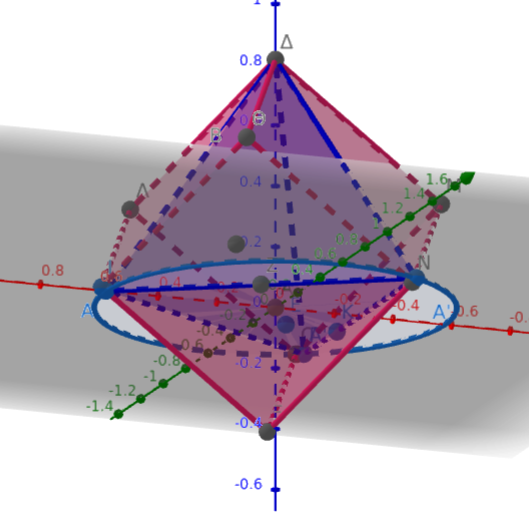
https://mathematica.gr/forum/viewtopic.php?f=179&t=72448&p=351756#p351756
Πόσο χαμηλά θα πέσω ;
Μέσα σε κύκλο ακτίνας 
, με βάση την μεταβλητή οριζόντια χορδή 
, σχεδιάζω ορθογώνιο 
με εμβαδόν : 
. Πόσο ψηλά και – κυρίως – πόσο χαμηλά μπορεί να βρεθεί η πλευρά 
;
Υπόδειξη : Μην αναζητήσετε τον γεωμετρικό τόπο της κορυφής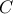 
! 
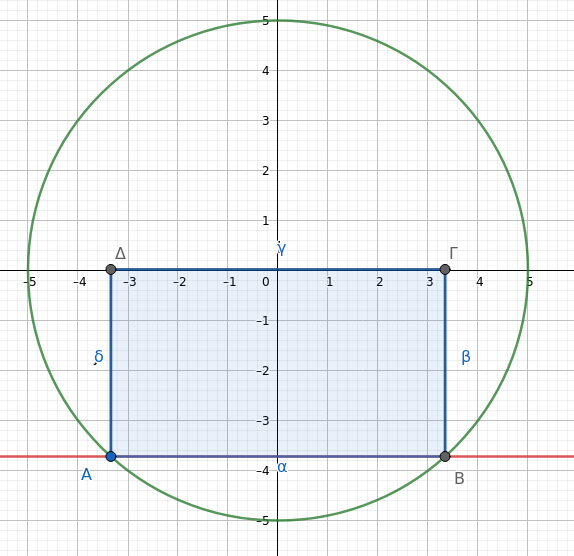
Γεωμετρική άσκηση
Του Σωκράτη Ρωμανίδη
https://eisatopon.blogspot.com/2022/10/p-q-r-s.html
Στο παρακάτω σχήμα τα σημεία \(P, Q, R\) και \(S\) είναι σημεία σε κύκλο με κέντρο Ο. Η ευθεία UV είναι εφαπτομένη στον κύκλο στο σημείο P.Τα τμήματα PR και OS τέμνονται στο T και \( \widehat{PQW} =106^o\) και \( SP =SR \). Να υπολογισθούν οι γωνίες:
i) \( \widehat{PSR} \) ii) \( \widehat{R_3}\) iii) \( \widehat{P_5}\) iv) \( \widehat{O_1} \) v) \( \widehat{P_3}\)
πρόβλημα Γεωμετρίας
Θεώρημα Poncelet-Steiner
Γεωμετρικές κατασκευές με κανόνα και «σκουριασμένο» διαβήτη !
https://en.wikipedia.org/wiki/Poncelet%E2%80%93Steiner_theorem
Κατασκευή Παράλληλης από σημείο εκτός ευθείες προς δοσμένη ευθεία ΑΒ με δοσμένο μέσο του ΑΒ
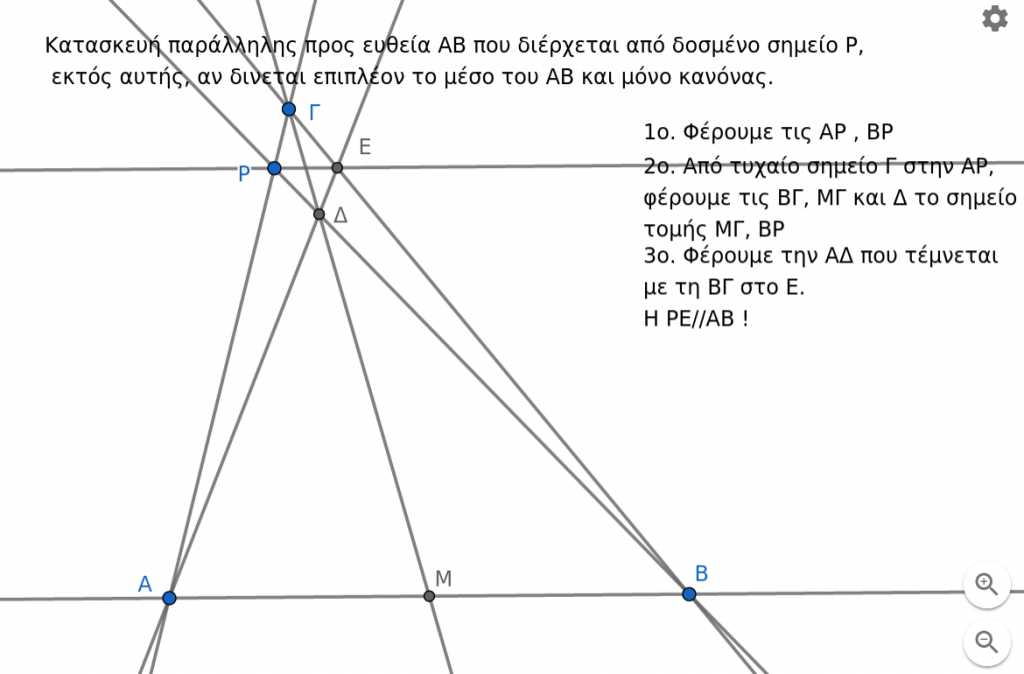
Δείτε την κατασκευή στο παρακάτω gifακι:
https://www.geogebra.org/geometry/mxvcjzuw?embed
Κατασκευή κάθετης προς ευθεία από δοσμένο σημείο.
https://www.geogebra.org/classic/zzquanan
Κατασκευή steiner ευθυγράμμου τμήματος σε δοσμένη ευθεία, με το μέσο του
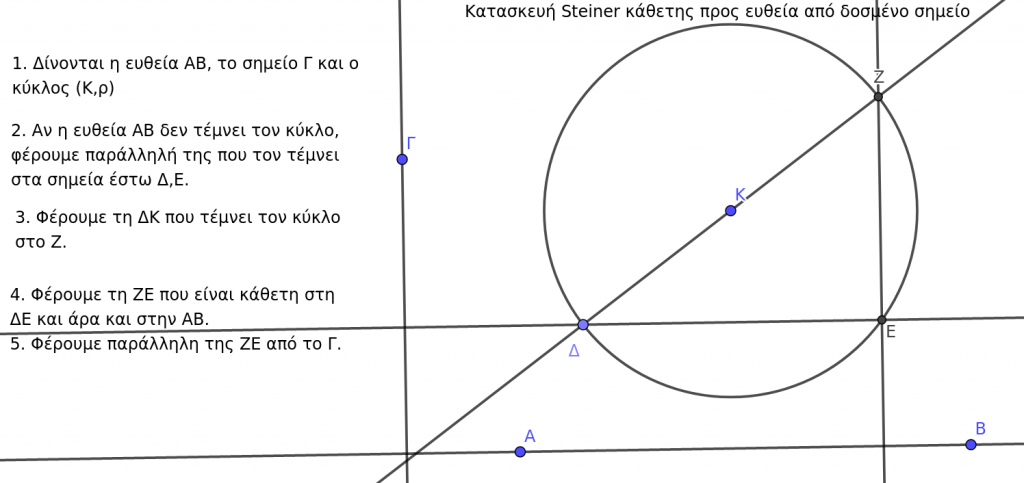
https://www.geogebra.org/classic/becnhq6t
Κατασκευή Steiner παράλληλης από δοσμένο σημείο σε διάμετρο δοσμένου κύκλου.
https://www.geogebra.org/classic/mftdyqv3
The Poncelet-Steiner theorem says
Everything you can construct with a straightedge and a compass you can construct by the straightedge alone, provided you are given a circle and its center.
Motivated by Mascheroni’s result ↑ J.V.Poncelet conjectured this results in 1812��[1]��and it was proved by J.Steiner [2]��in 1833.
It can be shown that the constructions cannot be done by straightedge alone [3] . By the straightedge alone only the so called linear constructions can be done. For instance, using the straightedge alone, without a circle given, is not sufficient to construct square roots. Even simpler constructions as to half a straight line segment are impossible by the straightedge alone. Another example is the result known as Steiner’s theorem:
Steiner’s Theorem: It is impossible to find the center of a given circle with the straightedge alone.
The basic idea of the following proof goes back to Hilbert. If such a construction would be possible, then it would be preserved by projective transformations.��This due to the basic properties of projective transformation which preserve lines, objects constructible by the straightedge. On the other hand, the circle as a conic section is transformed to a conic section in general.��Even worse, the conjugate diameters 1 of a conic section pre-image may not be transformed to the conjugate diameters of the image. Consequently, the center of circle is not projected to the center of the image.
