Κατηγορία: Α λυκείου
Όλα τα τρίγωνα είναι ίσα μεταξύ τους!
Ταξινόμηση Τετραπλεύρων
Συνευθειακά σημεία Πότε και πώς
3o Μαθη(μα)τικό Συμπόσιο 2025
Συνάδελφοι, μαθητές και γονείς ελάτε να διαδράσουμε με όμορφα Μαθηματικά Παιχνίδια, Ιστορίες και Θεατρικά
το Σάββατο 5 Απριλίου 2025 ώρα: 09:30 – 14:30 στο 6ο Γυμνάσιο Αθηνών, Ζεύξιδος και Πυθέου, Αθήνα, πλησίον μετρό ΣΥΓΓΡΟΥ-ΦΙΞ.
Τετράπλευρο σε τετράπλευρο Διερευνητική εργασία
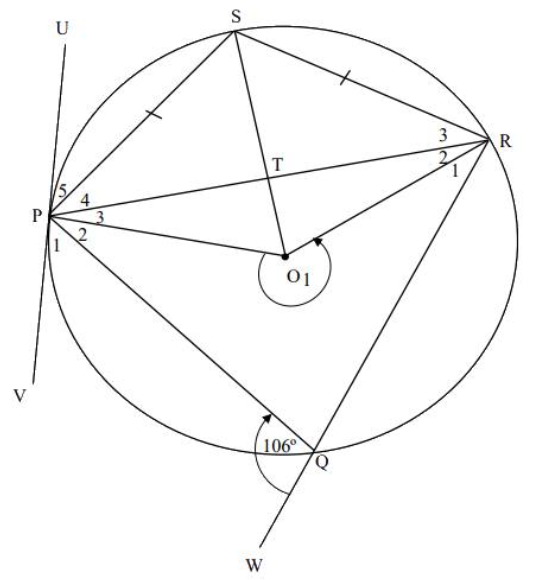
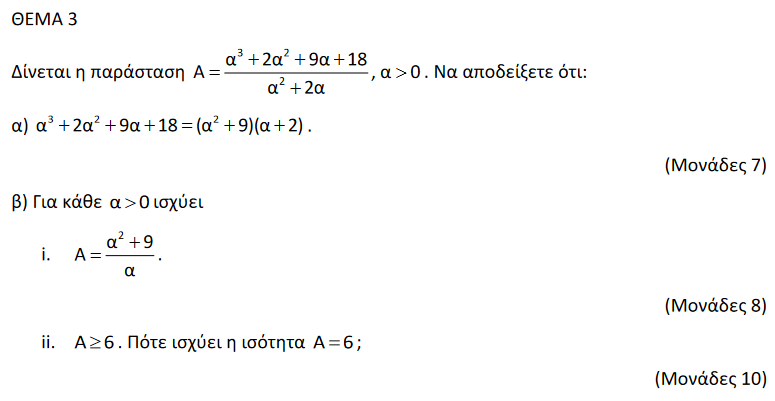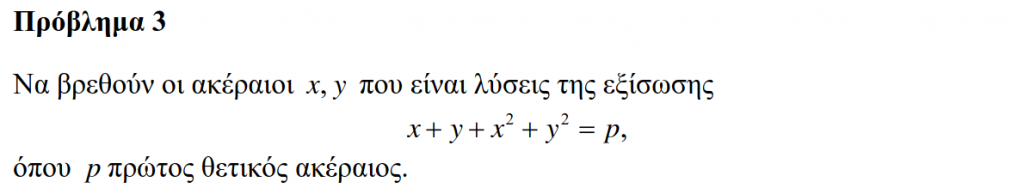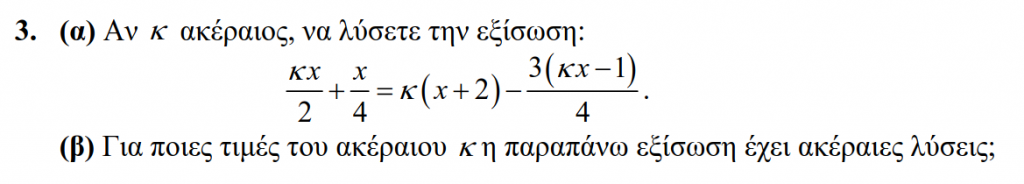Τετράπλευρο σε τετράπλευρο Περιγραφή:
Δίνεται το τετράπλευρο ΑΒΓΔ, όπως στο σχήμα.
Αν Ε, Ζ, Η, Θ μέσα των αντιστοίχων πλευρών του, τότε:
α) Βρείτε όσες περισσότερες παρατηρήσεις μπορείτε
β) Αποδείξτε ή απορρίψτε όσες από τις παρατηρήσεις γράψατε στο προηγούμενο ερώτημα.
Διερευνώντας τον περιγεγραμμένο κύκλο ενός τετραπλεύρου
Μπορείτε να βρείτε κύκλο ο οποίος να διέρχεται από τρία σημεία στο επίπεδο; Υπάρχουν προϋποθέσεις;
Μπορείτε να βρείτε κύκλο ο οποίος να διέρχεται από τέσσερα σημεία στο επίπεδο; Υπάρχουν προϋποθέσεις;
Η παρουσίαση:
Δείτε το φύλλο εργασίας εδώ.
Δείτε την τάξη εργασίας geogebraclassroom εδώ.
Δείτε τη διαδραστική ταινία για τα εγγράψιμμα τετράπλευρα εδώ:
Αποδεικνύοντας ανισότητες 2016
Εισαγωγή στις βασικές ανισότητες και στις αποδείξεις τους με χρήση των γνωστών από το σχολείο.
Το φυλλάδιο δεν έχει επικαιροποιηθεί από το 2016 και υπάρχουν μερικά τυπογραφικά λάθη, ως προς το πρόσημο κάποιων αριθμών.
Αλυκ14713Αλγ3
Μηδέν: Τίποτα ή τα Πάντα;
των συναδέλφων Χρήστου Κυριαζή και Λευτέρη Πρωτοπαπά, από το συνέδριο της ΕΜΕ 2014